Q.1 In fig.6.17,(i)and(ii),DE || BC. find EC in(i)and AD in (ii)
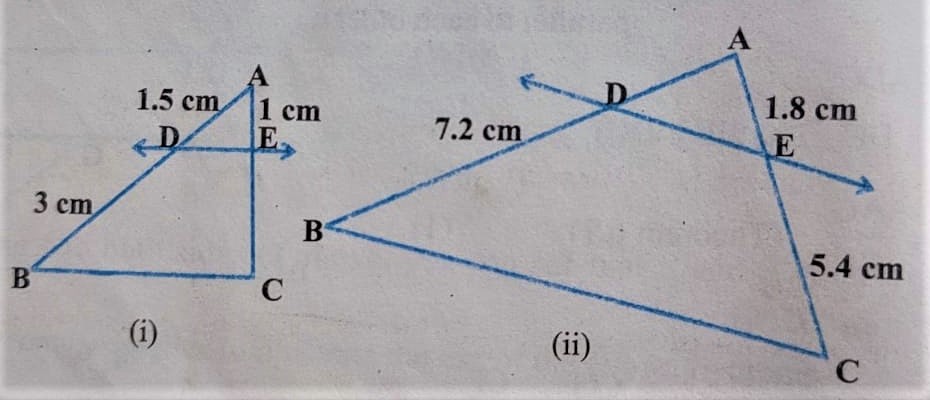
(i)
Solution:
According to figure (i) in ΔABC
DE || BC { given }
By B.P.T. [ In a triangle if a line is parallel to one side divides remaining to sides in equal ratio ]
AD / BD = AE / EC
1.5/3 = 1/EC
⇒1.5EC = 3
⇒ EC = 3/1.5
⇒ EC = 30/15
⇒ EC = 2cm
(ii)
Solution:
According to Figure (ii)
in ΔABC
DE || BC { given }
by B.P.T. [ In a triangle if a line is parallel to one side, divide the remaining two sides in an equal ratio ]
AD/BD = AE/CE
⇒ AD/7.2 = 1.8/5.4
⇒ AD = 1.8×7.2×10/5.4×10×10
⇒ AD = 24/10
⇒ AD = 2.4 cm
Q.2 E and F are points on the sides of PQ and PR respectively of a Δ PQR. For each of the following cases , state whether
EF || QR.
(i) PE = 3.9cm, EQ = 3cm, PE = 3.6cm and FR = 2.4cm
Solution:
PE/QE = PE/FR
⇒ 3.9/3×10 = 3.6×10/2.4×10
⇒ 13/10 ≠ 3/2 [ Sides of ration are not equal ]
Hence EF is not || to QR.
(ii) PE=4cm, QR=4.5cm, PF=8cm and RF=9cm
Solution:
PE/QE = PF/RF
⇒ 4/4.5 ≠ 8/9 [ Sides of ration are not equal ]
⇒ Hence EF is not parallel to QR
(iii) PQ= 1.28cm, PR=2.56cm, PE=0.18cm and PF=0.36cm.
Solution:
IF PQ/PE = PR/PF
⇒ 1.28/0.18 = 2.56/0.36
⇒ 128×100/18×100 = 256×100/36×100
⇒ 128/18 = 256/36
⇒ 64/9 = 64/9 [ Sides of ratio are not equal ]
by Converse of BPT
EF is Parallel to QR
Q.3 In fig. 6.18 if LM || CB and LN || CD, prove that
AM/AB = AN/AD
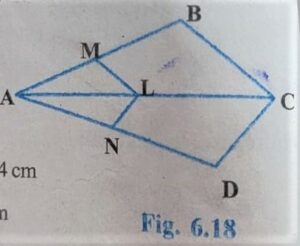
Solution:
According to Figure:-
In Δ ABC
LM || CB { given }
BY B.P.T. [ In a triangle if a line is parallel to one side divide the remaining two sides in an equal ratio ]
AM/AB = AL/AC ———(i)
Similarly
In Δ ADC
LN || CD
By B.P.T.
AN/AD = AL/AC ———-(ii)
AM/AB = AN/AD
Hence proved
Q.4 In fig. 6.19, DE || AC and DF || AE Prove that BF/FE = BE/EC
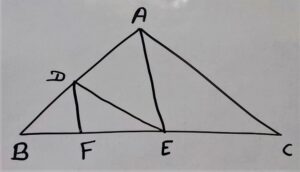
Solution:
In Δ ABC
DE || AC
BD/AD = BE/CE [∴by B.P.T.] ——–(i)
Similarly
In Δ ABE
DF || AE
BD/AD = BF/EF ———(ii) [ by B.P.T ]
by comparing equation (i) and equation(ii)
We get
BE/CE = BF/EF
⇒ BE/EC = BF/FE
Hence Proved
Q.5 In fig. 6.20, DE || OQ and DE || OR. show that EF || QR.
Given:
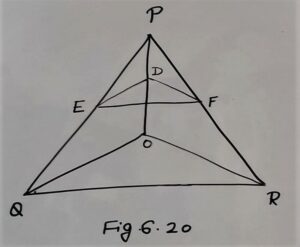
DE || OQ
DE || OR
To Show:
EF II QR
Solution:
In ΔPOQ
DE || OQ
PE/EQ = PD/DO ———(i) [ ∴ by B.P.T. ]
In ΔPOR
DF || OR
PE/FR = PD/DO——–(ii) [∴ by BPT ]
by equation (i) and equation (ii)
PE/EQ = PF/FR
By converse of BPT
EF || OR
equation (i) and equation (ii)
Q.6 In Fig. 6.21 A, B, and C are points on OP, OQ, and OR respectively such that AB || PQ and AC || PR Show that BC || QR
Given:
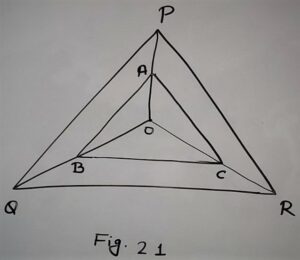
A, B, and C are lies on sides OP, OQ, and OR respectively
AB || PQ,
AC || PR
Solution:
In Δ PQR
AB || PQ [∴given]
by B.P.T.
PA/AO = QB/BO ———-(i)
Similarly
In Δ POR
AC || PR [∴ given ]
by B.P.T.
PA/AO = RC/CO ———-(ii)
Q.7 Using Theorem 6.1, prove that a line drawn through the mid-point of one side of a triangle parallel to another side bisects the third side.
Given:
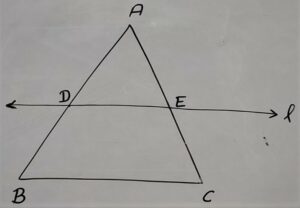
In ΔABC
D is the midpoint of side AB
i.e. AD=DB
BC II Line l [BC parallel to line l]
Line l intersects AC at E.
To Prove:
E, Mid-point of AC
Proof:
Here According to the figure
In ΔABC
D is the mid-point of AB
AD = DB [Given]
AD/BD = 1 …………(i)
DE II BC [Given]
∴ AD/DB = AE/EC [By BPT]
⇒ 1 = AE/EC [From equation (i)]
∴ AE = EC
∴ E is the mid-point of AC.
Q.8 Using Theorem 6.2, prove that the line joining the mid-points of any two sides of a triangle is parallel to the third side.
Given:
In ΔABC
D = Mid-point of AB
E = Mid-point of AC
Join D to E
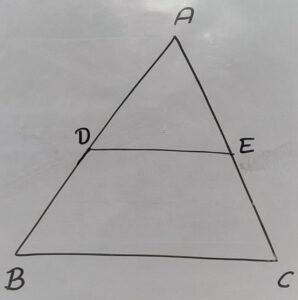
To Prove:
DE II BC
Proof:
According to Figure
In ΔABC
D is the mid-point of AB [given]
∴ AD=DB
⇒ AD/DB=1 ……………………..(i)
E is the Mid-point of AC [Given]
∴ AE=EC
⇒ AE/EC=1 ……………………….(ii)
From Equation(i) and Equation(ii)
We get
AD/DB = AE/EC
By Converse of BPT
DE II BC
Hence Proved.
Q.9 ABCD is a trapezium in which AB || DC and its diagonals intersect each other at point O. Show that
AO/BO = CO/DO
Given:
In trapezium ABCD
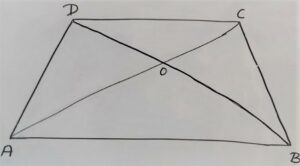
AB || DC
To Show:
AO/BO = CO/DO
Construction:
EO || AB
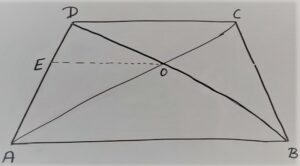
Proof:
In Δ ABD
EO || AB [∴by const.]
By BPT
AE/ED = BO/OD ———(i)
In Δ ACD
AB || DC [∴ given ]
EO || AB [∴ By construction ]
∴ DC || EO
AE/ED = AO/CO ——-(ii)
by comparing equation(i) and equation(ii)
BO/OD = AO/CO
⇒ CO/DO = AO/BO
⇒ AO/BO = CO/DO
Hence Proved
Q.10 The diagonals of a quadrilateral ABCD intersect each other at point O such that AO/BO = CO/DO. Show that ABCD is a trapezium.
Given:
In Quadrilateral ABCD

AO/BO = CO/DO
⇒ AO/CO = BO/DO ——–(i)
To Show:
ABCD is a trapezium
Construction:
EO || AB

Proof:
In Δ ABD
EO || AB [∴by construction]
by B.P.T.
AE/DE = BO/DO ———(ii)
by comparing equation(i) and equation(ii)
We get
AO/CO = AE/DE ——-(iii)
In Δ ACD
AO/CO = AE/DE [∴From Equation (iii) ]
By the converse of BPT
EO || DC
∴ EO || AB [∴ by construction]
∴ AB || DC
Hence pair of one side are parallel of Quadrilateral ABCD .
∴ Quadrilateral ABCD is a trapezium .
Hence proved.
